Comparing Brake Pressure to Braking G Force - or - Reviewing Braking Inputs without Brake Pressure Data
Comparing Brake Pressure to Braking G Force - or - Reviewing Braking Inputs without Brake Pressure Data
In conducting data analysis of braking, people often assume that they need to have brake pressure sensor data. While this is ideal, it's often complex and expensive to obtain. Alternatively, and admittedly not a perfect substitute, we can look at deceleration force (negative longitudinal G force).
One consideration is that effective braking force (the aforementioned negative longitudinal G force) is that there will be a slight delay compared to brake pressure is initially applied because the deceleration happens after the brakes are applied. This becomes more evident in looking in detail at the exact shape of the curves of brake force versus brake pressure.
I usually filter out the first -0.3G of deceleration as this is approximately what we see with engine braking alone when there's not a lot of aerodynamics at play (below 100 mph there's a little, below ~50mph, virtually none. If you're using a 2-stroke bike or one that has very little engine braking, you might want to change that threshold.
Assuming that the driver/rider is already exhibiting a good level of competence, looking at deceleration force is a reasonable substitute for exact brake pressure. Ideally we have both but it's not absolutely necessary for most amateur riders/drivers.
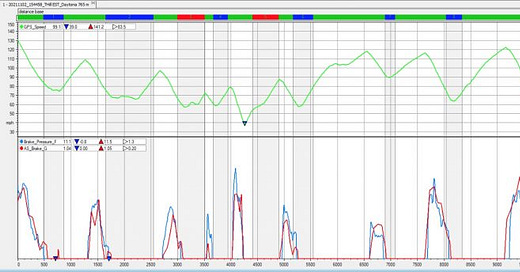
In the below example, the first graph is the speed trace (GPS speed) and the second graph has brake pressure (front brakes only) and negative longitudinal G force.
Once you have deceleration force to review, you can also begin to look at things like trail braking by factoring in a math channel looking at lean angle + longitudinal deceleration. While I'm still reviewing these intricacies, you can read up on it with someone with more experience than I can claim dataMC.org. In this example, the top chart is the GPS speed trace, the 2nd is braking force, the third is trail braking (continuing to hold the brake past tip in, ideally to the slowest point of the corner). Keep in mind this is absolute G force, so it does not account for camber in the road surface, much like dashes of motorcycles that indicate lean angle.
